A simpler way to predict bolt preload

Engineers must frequently calculate the bolt preload torque needed to securely hold parts together without overstressing joints. They mainly rely on torque equations and torque tables, time-consuming tools that were developed more than a half-century ago. Here is a user-friendly alternative.

Generalized torque coefficient
Bolt-preloading torque, T, consists of three components that share a common factor.

T = (C_L + C_t + C_c)(DF_i),

where torque coefficients for lifting, thread friction, and collar friction, respectively, are:

C_L = L/2πD,

C_c = µ_cD_c/2D

Denoting the overall torque coefficient as C = C_L + C_t + C_c, the equation reduces to T = C(DF_i).

This equation is similar to the classical approximation T = 0.2 DF_ithat applies to coefficient of friction (c.o.f.) μ_c = μ_t = 0.15 for small screws. Torque coefficient, C, however, is generalized to embrace all screw sizes and c.o.f.s. Only two factors affect the equations — bolt size and friction — provided μc = μt and D_c = 1.25D. The generalized torque-coefficients table lists values for C for selected screw sizes and c.o.f.s. The data directly link torque and clamping force, thus eliminating the need to toil over the torque equation.

For example, let’s determine the clamping forces produced from 70 to 80 lbf-in. torque on a 0.250-28UNF screw. Assume a c.o.f. of 0.10 to 0.15 for both threads and nut collar.

From the table, torque coefficients for a 0.250-in.-diameter screw are C_max = 0.195915 at maximum friction µ_max = 0.15; and C_min = 0.138035 at minimum friction µ_min = 0.10.

F_imin = -T_min/C_maxD = 1,429 lbf, and
F_imax = T_max/C_minD = 2,318 lbf.

Note that data in the table are based on the assumptions that, µ_c= µ = µt and D_c =1.25 D. If either of these conditions is violated, adjust the torque coefficient using:

C’ = C – 0.625µ + 0.5µ_cD_c/D.

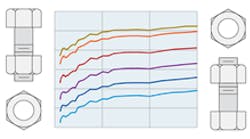
The accompanying “Torque-coefficients” graph puts values from the table in perspective. The classical equation T = 0.2 DF_iis reasonably accurate for c.o.f. = 0.15 and size 0.25 in. and smaller screws; that is, C ≈ 0.2. Bolt size has a moderate effect on C but the effect of c.o.f. is substantial. Average torque coefficients for all sizes in the generalized table are close to a straight line: C = 0.02 + 1.17μ. Therefore, engineers can use linear interpolation to determine C across c.o.f.s for a specific bolt size. Typical values of c.o.f. are about 0.1 to 0.2.

Universal torque table

Applied torque concurrently produces both tensile and shear stresses in a bolt. Shear stress originates from the bolt twisting torque T_b where:

T_b = C_bT/C and
C_b = C – C_c = C – 0.625µ.

Tensile stress, σ_t = F_i/A_t = T/CDA_t.

Shear stress τ = T_b(d_r/2)/(πdr⁴/32) = 16Tb/πdr³ = (16/πdr³)(Cb/C)T.

Engineers cannot judge a design’s safety by comparing this two-dimensional stress with material tensile strength. Conventional methods combine tension and shear stresses into an equivalent stress and compare it with material strength. A general notion is that if this equivalent stress reaches the material strength, the part fails. The maximum distortion energy criterion of failure stipulates that inelastic failure occurs when von Mises stress approaches the material yield stress.

For most bolt materials, von Mises stress criteria applies. Von Mises stress during preloading is:

σ_v = (σ_t² + 3τ²)^0.5.

It is straightforward, though laborious and error-prone, to calculate σ_v from T using the σ_t, τ, and σv equations, but there is no explicit method to calculate T for a given σ_v. Engineers traditionally use a trial-and-error method: Initially guess a T to calculate σ_v; compare the result with the target value; and if unsatisfactory, try again.

This is one of the most taxing tasks in bolt-stress analysis. And it happens to be important because engineers are often challenged to determine a torque that uses every bit of allowable stress based on material strength and the safety factor.

The following embedding technique circumvents the hurdle. First, embed τ into σ_t.

The proportional constant ξv is a multiplier to convert σ_t to σ_v, while τ is embedded into σ_t. Second, substitute

T = C(DF_i) and Fi = A_tσ_t.

The proportional constant, Kv, depends on bolt size and c.o.f., and could be tabulated in the same way as torque coefficients. Alternatively, universal torque values Tu can be tabulated for a fixed value of von Mises stress σu based on the relation Tu = Kvσu. The universal torque table does just that for σu = 100 ksi. This method replaces the traditionally laborious trial-and-error preload calculations with bidirectional data scaling:

σ_v = σ_u(T/T_u), or T = T_u(σ_v/σ_u).

Here’s how it works. Say a 0.250-28UNF screw is preloaded to a maximum stress of 90 ksi. Assume c.o.f. ranges from 0.10 to 0.15 and torque tolerance is 0 to –15%. Determine the maximum and minimum torques and clamping forces. Von Mises stress in the universal torque table is σu = 100 ksi; for a 0.250-28UNF screw and c.o.f. = 0.10, T_u = 102 lbf-in.; and maximum allowable von Mises stress at preloading is σ_max = 90 ksi.

Calculate maximum and minimum preload torques from:

T_max = T_u(σ_max/σ_u) = 102(90/100) = 91.8 lbf-in. and
T_min = (1 – 0.15)T_max = 0.85 × 91.8 = 78 lbf-in.

For a 0.250-28UNF screw, from the generalized torque coefficients table, C_max = 0.195915 for c.o.f. = 0.15 and C_min = 0.138035 for c.o.f. = 0.10.

Maximum and minimum clamping forces are:

F_imax = T_max/C_minD = 91.8/(0.138035 × 0.25) = 2,654 lbf,
F_imin = T_min/C_maxD = 78/(0.195915 × 0.25) = 1,593 lbf.

The universal torque and torque coefficient link preload torque, stress, and clamping force. Given any one, determine the other two through simple math.

Additional concerns
Clamping capability. Determine the theoretical upper bound of clamping capability by pushing von Mises stress at assembly to the yield stress of the material.

Let σ_v = σ_y , because σ_v = ξ_vσ_t. Therefore, σ_t/σ_y = F_i/A_t.σ_y = 1/ξ_v. The “Clamping force” graph shows the clamping-to-yield-force ratio, F_i/A_t.σ_y, depends on both bolt size and c.o.f. For example, for a 0.25-28UNF screw with c.o.f. = 0.15, the upper bound of clamping force is about 72% of the bolt yield strength in the threaded section. This percentage changes for other bolt sizes and c.o.f.s. Therefore, it would be fruitless to set one clamp/yield force ratio or tensile/yield stress ratio and hope to apply it uniformly. In contrast, one von Mises/yield stress ratio, σ_v/σ_y, applies to all sizes, c.o.f.s, and materials. In actual designs, the ratios are even smaller because of the safety factor.

Adjustment for collar friction. Torque values in the universal torque table are based on assumptions that µ_t = µ_c and D_c= 1.25D. If one of these conditions is violated, first calculate C’ as shown in the previous torque-coefficient section and scale the torque accordingly. Adjusted torque is T’ = T(C’/C).

Adjustment for mild steel bolts. For lower-strength materials, the maximum shear-stress failure criterion applies. This states that when the maximum shear stress reaches half the material yield stress, the part fails. That leads to equivalent stress during preloading:

σ_m = (σ_t² +4τ²)^0.5

Torque needs to be scaled down to maintain the same margin of safety.

For the screw sizes in the universal torque table, the torque reduction factor is: 0.98 > (ξv / ξm) > 0.88 for 0.08 ≤ µ ≤ 0.30.

Torque tables. Traditional torque tables typically list bolt-preload torques for a specific bolt-material strength and surface condition. It’s not uncommon for a project to require more than a dozen torque tables. Those tables are suitable for assembly shops but ineffective for stress analysis because they convey neither the von Mises stress produced from the torque nor the c.o.f. Engineers require the former to determine the margin of safety at assembly. And the latter is a key parameter to calculate stress, clamping force, and so on. The information must have been used to develop the torque tables but is not passed along with the tables.

A universal torque table, used in conjunction with torque coefficients, makes all essential information and processes transparent. Further, one universal torque table applies to all materials and surface conditions because preload control relates torque and von Mises stress irrespective of the material strength; and the c.o.f. is the core parameter in preload control irrespective of surface conditions.

Margins of safety. It’s good practice to limit preload to provide an adequate margin of safety. The safety margin = σy/(SFσv) – 1 > 0. SF is the safety factor at assembly, typically 1.1.

Design for preload in three steps:

1. Choose preload stress σ_v < σ_y/S_F.
2. Scale torque using the universal torque table.
3. Calculate clamping force using the torque coefficient.

Usually, it is not necessary to hold a portion of allowable stress in reserve to handle external loads because after preloading, there is no twisting shear and the bolt stress reduces from σ_v to σ_t by the ratio shown in “Clamping force” chart. Also, the bolt shares only a fraction of the external load. Nevertheless, engineers must account for the effects of external load.

Addressing external load. Combine the initial force on the bolt from preload torque with the external force to determine the total load under service conditions. Bolt load in service is F_b = F_i + Ï•Fe; and clamp load is F_c = -F_i + (1 – Ï•)F_e.

Determine the bolt load-sharing ratio, Ï•, from stiffnesses of the bolt and clamped members. Typical values of Ï• are 0.2 to 0.5. Use the F_b equation to determine the margin of safety for the bolt. Use the F_c equation to ensure that clamping force is sufficient to prevent joint leakage or separation. Generally, the clamping force has a margin of error resulting from tolerances in both c.o.f. and the wrench torque reading. Therefore, the F_b equation uses the maximum F_i, and the F_c equation uses the minimum Fi in absolute terms. Preloading control is complete only after the margins of safety for both bolt load and clamping force are satisfied for all service conditions.

© 2011 Penton Media, Inc.