Structural Properties of Bolted Joints

Sound designs of bolted joints rely on a good grasp of their structural behavior, and structural properties of preloaded bolted joints — including the bolt load-sharing ratio, separation load ratio, and joint stiffness — depend only on the stiffnesses of the bolt and clamped members. Nonetheless, bolted joints are rather complicated. Here’s how engineers can approximate bolt and clamped-member stiffnesses and derive structural parameters useful for design.

Bolts and clamped members can be modeled as bars connected in series for stiffness calculations. The stiffness of a bar with uniform cross-sectional area A and length L is related to its modulus of elasticity E = σ/ε, the ratio of stress and strain. If a force F elongates a bar by δ, then the bar stiffness is:

Bolt stiffness
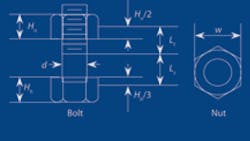
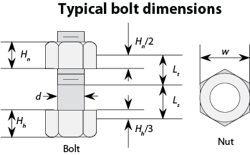
Bolts consist of shank and threaded sections, as shown. Stiffness of the shank is:

where A_s = πd²/4.

The threaded-section stiffness is:

where A_t = tensile-stress area of the screw.

The tensile-stress area of the screw can be found in handbooks and textbooks. Or calculate it based on the equation from ASME Standard B1.1-1989 (revision of ANSI B1.1-1982) for unified inch screw threads:

where D = basic major diameter. The basic pitch diameter d_2b = D – 0.75 H; H = height of a sharp V-thread or fundamental triangle, H = √3(P/2); and P = thread pitch. 1/P is the number of threads per inch.

Or determine the stress area of the screw based on the area of a circle with the average diameter, d_a, calculated from the basic pitch diameter, d_2b, and minimum minor diameter d_1m = D – 1.5 H.

The effective length, Lt, of the threaded section is from the interior end to the midpoint of the nut height. This assumes that bolt tensile force decreases linearly from the seating surface to the free surface of the nut. The effective length of the shank, Ls, includes one-third of the bolt-head height. This assumes bolt force diminishes linearly along the height of the bolt head starting at the mean area of the seating surface. Because the widths across flats of the bolt head are typically w = 1.5d, the mean diameter of stress area in bolt head is d_m = (d+w)/2.

Equivalent stiffness of the head in terms of the shank is

For studs with or without threaded inserts, include one-half of the thread-engagement length in the threaded-section length for stiffness calculations. These approximations are judgment calls. Other methods use d/4 in lieu of both H_n/2 and H_h/3. The series spring-connection method may be extended to bolts with multiple diameters.

Calculate total bolt stiffness, kb, from series connection of the section stiffnesses:

Clamped members Clamped members can be treated as a sleeve. The stress area of the hollow cylinder is:

A_c = π(d_o²– d_i²)/4

where d_o and d_i = outside and inside diameters, respectively. The clamped-member stiffness is:

k_c = A_cE_c/L_c.

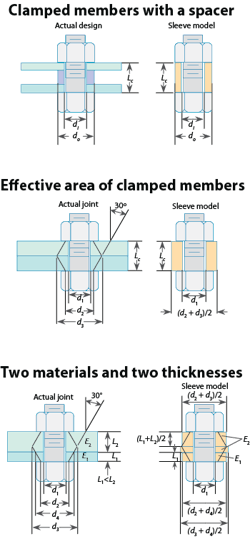
Calculate the clamped member stiffness of a solid structural connection using a double-cone load-path model, as shown in the graphic, “Effective area of clamped members.” A 30° cone angle is customarily used in industry. If clamped members have the same modulus of elasticity, the double cone shown is equivalent to the sleeve model.

The double-cone model can be modified to accommodate clamped members with different moduli of elasticity and thickness as shown in “Two materials and two thicknesses.” The stiffness of clamped members is calculated from series connections of three hollow cylinders shown in the sleeve model, assuming L1.

Gaskets and seals
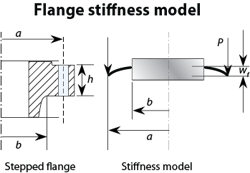
Some O-rings rely on internal pressure to entrap rubber in the groove for sealing. These seals may not be in the structural load path and can be ignored for stiffness calculations. In other cases an O-ring may dominate the compliance (inverse of stiffness) of clamped members. For O-rings with solid, hollow, or other cross sections, stiffness data should be translated to the clamped member stiffness for each bolt. For example, if it takes linear force f (force/length) to deform the seal by δ, and the joint has N bolts equally spaced on the bolt center circle with diameter D_c, then each bolt’s share of the spring rate of the seal is: k_s = πD_cf/Nδ.